Next: Sample Construction Up: Introduction to Rare Earth/Iron Previous: Bulk Properties Contents
The RFe![]() family of intermetallics have shown potential uses in a number of nano-scale applications such as magnetic media readheads and nanosensors and so their behaviour in thin films and other low-dimensional systems has become of great interest to fundamental and applied research. Going from bulk to a thin film increases the effect of otherwise negligible effects such as shape anisotropy, strain and interface effects.
family of intermetallics have shown potential uses in a number of nano-scale applications such as magnetic media readheads and nanosensors and so their behaviour in thin films and other low-dimensional systems has become of great interest to fundamental and applied research. Going from bulk to a thin film increases the effect of otherwise negligible effects such as shape anisotropy, strain and interface effects.
Previous work[29] on expitaxial Laves Phase DyFe![]() thin film samples highlighted the importance of expitaxial strain in perturbing the magnetic behaviour seen in bulk samples. In pure rare earths the anisotropy energy is roughly an order of magnitude greater than the magnetoelastic energy. RFe
thin film samples highlighted the importance of expitaxial strain in perturbing the magnetic behaviour seen in bulk samples. In pure rare earths the anisotropy energy is roughly an order of magnitude greater than the magnetoelastic energy. RFe![]() systems, however, show giant magnetostriction at room temperature. The magnetoelastic energies in these samples can be large enough to strongly influence the overall anisotropy energy and hence the magnetic easy axis.
systems, however, show giant magnetostriction at room temperature. The magnetoelastic energies in these samples can be large enough to strongly influence the overall anisotropy energy and hence the magnetic easy axis.
In an unstressed sample the dominant influence on the direction of the easy axis is the magnetocrystalline anisotropy energy, ![]() , given by[30]
, given by[30]
| (6.3) |
In the samples studied in this chapter there are strains caused by a difference in thermal contraction between film and substrate as the sample cools from the deposition temperature.[30] It is now necessary to take into account the magnetoelastic energy, ![]() , given by
, given by
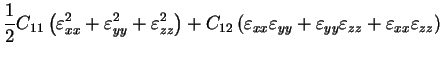 |
|||
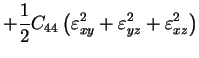 |
(6.5) |
The main strain in the samples is a negative shear[30] of
![]() . This simplifies Equation 6.4 to
. This simplifies Equation 6.4 to
![]() . The total energy,
. The total energy, ![]() , is thus
, is thus
| (6.6) |
Dr John Bland, 15/03/2003