Next: Bulk Properties Up: RFe Laves Phase Thin Previous: RFe Laves Phase Thin Contents
The Lanthanides are the group of elements with atomic numbers between 57 and 71. They are often accompanied by yttrium as it has similar chemical characteristics and are termed Rare Earths due to the difficulty with which they are chemically isolated. This difficulty arises from the typical atomic configuration of a rare earth[13], [Xe]
![]() . The
. The ![]() shell is partially filled whilst the outer valence electrons are much the same in nature throughout the series, giving similar chemical characteristics to all fifteen elements. The partially filled
shell is partially filled whilst the outer valence electrons are much the same in nature throughout the series, giving similar chemical characteristics to all fifteen elements. The partially filled ![]() shells in rare earths lead to magnetic properties in a similar manner to the partially filled
shells in rare earths lead to magnetic properties in a similar manner to the partially filled ![]() shell in transition elements.
shell in transition elements.
In an intermetallic of a rare earth and a transition element the spatial extent of the ![]() wavefunction is highly localised giving very weak interactions between rare earth atoms. In such compounds where the transition element carries no magnetic moment this gives rise to low ordering temperatures,[24] eg T
wavefunction is highly localised giving very weak interactions between rare earth atoms. In such compounds where the transition element carries no magnetic moment this gives rise to low ordering temperatures,[24] eg T
![]() for DyNi
for DyNi![]() . Any interactions must take place indirectly through such mechanisms as spin polarization of the
. Any interactions must take place indirectly through such mechanisms as spin polarization of the ![]() -conduction electrons[24]. The localised
-conduction electrons[24]. The localised ![]() moments are affected by the polarisation produced by
moments are affected by the polarisation produced by ![]() moments elsewhere in the lattice and can orient themselves accordingly. This polarization is not uniform in space and has an oscillitatory form as given by the RKKY interaction (see Section 5.4).
moments elsewhere in the lattice and can orient themselves accordingly. This polarization is not uniform in space and has an oscillitatory form as given by the RKKY interaction (see Section 5.4).
The transition element, however, has much stronger and more long range interatomic interactions due the large spatial extent of the ![]() electron wavefunctions. The wavefunctions of neighbouring atoms overlap producing
electron wavefunctions. The wavefunctions of neighbouring atoms overlap producing ![]() -electron energy bands rather than levels. The strong exchange interactions between the
-electron energy bands rather than levels. The strong exchange interactions between the ![]() electrons can produce unequal numbers of spin-up and spin-down electrons. Relative differences in the density of states of
electrons can produce unequal numbers of spin-up and spin-down electrons. Relative differences in the density of states of ![]() electrons produces a net moment given by
electrons produces a net moment given by
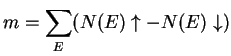 |
(6.1) |
The magnetic interaction between the rare earth and transition element has a strength intermediate between that of the two cases discussed above. The rare earth and transition element sublattices couple antiparallel in the case of a heavy rare earth (eg Holmium) and parallel in the case of a light rare earth (eg Praseodymium). The ![]() moments align antiferromagnetically with the spin-moment of the rare earth and the difference in coupling between the light and heavy rare earth cases is attributed to the total angular momentum of a rare earth being
moments align antiferromagnetically with the spin-moment of the rare earth and the difference in coupling between the light and heavy rare earth cases is attributed to the total angular momentum of a rare earth being ![]() for light rare earths and
for light rare earths and ![]() for heavy rare earths.[24]
for heavy rare earths.[24]
Another way in which the rare earth and transition element differ is in the strength and types of magnetic anisotropy they display. Dipolar interactions are proportional to the square of the moment, the pair energy of a magnetic dipole interaction given by[10]
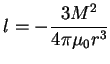 |
(6.2) |
The dominant contribution to anisotropy in these intermetallics is due to the effects of the crystal field on the rare earth's ![]() wavefunction. Rare earths have very strong single-ion magnetocrystalline anisotropy (see Section 5.2), essentially the interaction between orbital state and the crystal field. In bulk materials rare earth metals require applied fields of hundreds of kOe to overcome the anisotropic forces, compared to a few kOe for a transition element such as metallic Iron.
wavefunction. Rare earths have very strong single-ion magnetocrystalline anisotropy (see Section 5.2), essentially the interaction between orbital state and the crystal field. In bulk materials rare earth metals require applied fields of hundreds of kOe to overcome the anisotropic forces, compared to a few kOe for a transition element such as metallic Iron.
Intermetallics of rare earths and transition elements can combine the quite different natures of the two types of material via the antiferromagnetic coupling between the ![]() moment and
moment and ![]() spin-moment. The rare earth can provide strong magnetocrystalline anisotropy, a large magnetic moment per atom and a large degree of magnetostriction (see Section 5.3), and this is combined with the strong magnetic exchange coupling of the
spin-moment. The rare earth can provide strong magnetocrystalline anisotropy, a large magnetic moment per atom and a large degree of magnetostriction (see Section 5.3), and this is combined with the strong magnetic exchange coupling of the ![]() moments. Using particular elements and compositions we can engineer magnetic systems with specific properties for applications such as permanent magnets and recording media and readheads. The similar chemical properties but varying magnetic properties of the rare earths across the entire series also offers benefits for research as often the same compositions of intermetallic can be produced with all the rare earths but with widely varying physical properties allowing the investigation of fundamental properties such as spin and angular momentum under different conditions.
moments. Using particular elements and compositions we can engineer magnetic systems with specific properties for applications such as permanent magnets and recording media and readheads. The similar chemical properties but varying magnetic properties of the rare earths across the entire series also offers benefits for research as often the same compositions of intermetallic can be produced with all the rare earths but with widely varying physical properties allowing the investigation of fundamental properties such as spin and angular momentum under different conditions.